DREAM TO CONTROL: LEARNING BEHAVIORS BY LATENT IMAGINATION
Presented by
Bowen You
Introduction
In the general reinforcement learning framework, one typically trains an agent to learn complex behaviors. Intelligent agents are able to accomplish complex tasks that have never been seen in prior experience. One way to achieve this is by building a representation of the world based on past experiences. The authors propose an agent that learns long-horizon behaviors purely by latent imagination and outperforms previous agents in terms of data efficiency, computation time, and final performance.
Preliminaries
This section aims to define a few key concepts in reinforcement learning. In the typical reinforcement problem, an agent interacts with the environment. The environment is typically defined by a model that may or may not be known. The environment may be characterized by its state [math]\displaystyle{ s \in \mathcal{S} }[/math]. The agent may choose to take actions [math]\displaystyle{ a \in \mathcal{A} }[/math] to interact with the environment. Once an action is taken, the environment returns a reward [math]\displaystyle{ r \in \mathcal{R} }[/math]as feedback.
The actions an agent decides to take is defined by a policy function [math]\displaystyle{ \pi : \mathcal{S} \to \mathcal{A} }[/math]. Additionally we define functions [math]\displaystyle{ V_{\pi} : \mathcal{S} \to \mathbb{R} \in \mathcal{S} }[/math] and [math]\displaystyle{ Q_{\pi} : \mathcal{S} \times \mathcal{A} \to \mathbb{R} }[/math] to represent the value function and action-value functions of a given policy [math]\displaystyle{ \pi }[/math] respectively.
Thus the goal is to find an optimal policy [math]\displaystyle{ \pi_{*} }[/math] such that \[ \pi_{*} = \arg\max_{\pi} V_{\pi}(s) = \arg\max_{\pi} Q_{\pi}(s, a) \]
Feedback Loop
Given this framework, agents are able to interact with the environment in a sequential fashion, namely a sequence of actions, states, and rewards. Let [math]\displaystyle{ S_t, A_t, R_t }[/math] denote the state, action, and reward obtained at time [math]\displaystyle{ t = 1, 2, \ldots, T }[/math]. We call the tuple [math]\displaystyle{ (S_t, A_t, R_t) }[/math] one episode. This can be thought of as a feedback loop or a sequence \[ S_1, A_1, R_1, S_2, A_2, R_2, \ldots, S_T \]
Motivation
In many problems, the amount of actions an agent is able to take is limited. Then it is difficult to interact with the environment to learn an accurate representation of the world. The proposed method in this paper aims to solve this problem by "imagining" the state and reward that the action will provide. That is, given a state [math]\displaystyle{ S_t }[/math], the proposed method generates \[ \hat{A}_t, \hat{R}_t, \hat{S}_{t+1}, \ldots \]
By doing this, an agent is able to plan-ahead and perceive a representation of the environment without interacting with it. Once an action is made, the agent is able to update their representation of the world by the actual observation.
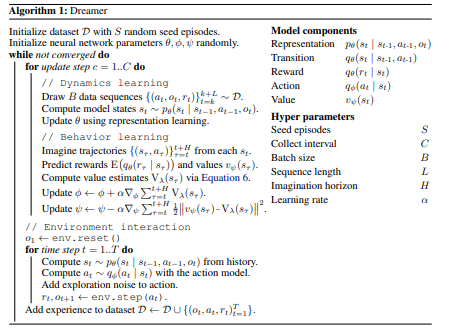
Dreamer
The authors of the paper call their method Dreamer. It consists of:
- Representation [math]\displaystyle{ p_{\theta}(s_t | s_{t-1}, a_{t-1}, o_{t}) }[/math]
- Transition [math]\displaystyle{ q_{\theta}(s_t | s_{t-1}, a_{t-1}) }[/math]
- Reward [math]\displaystyle{ q_{\theta}(r_t | s_t) }[/math]
- Action [math]\displaystyle{ q_{\phi}(a_t | s_t) }[/math]
- Value [math]\displaystyle{ v_{\psi}(s_t) }[/math]
where [math]\displaystyle{ \theta, \phi, \psi }[/math] are learned neural network parameters. The proposed algorithm is described below.