Phase speed is perpendicular to group speed
Let us assume that a fluid is inviscid, linear, non-diffusive, Boussinesq, and consists of motion independent of the  coordinate. The Navier-Stokes equations can therefore be written using the stream-function,
coordinate. The Navier-Stokes equations can therefore be written using the stream-function,  , as
, as
 .
.
Making the wave ansatz,  , where
, where  and
and  , the dispersion relation is
, the dispersion relation is

where  .
.
The phase speed is thus
 .
.
The group speed is

Therefore we find that the group velocity is perpendicular to the phase velocity!

This is completely counterintuitive from our experiences with surface waves, sound waves, and in fact most other waves, where the energy propagates in the same direction as the phase. The Saint Andrew's Cross experiment is the classic example demonstrating this. See Kundu for pictures and further elaboration.
Waves in a channel
Consider two-dimensional flow between boundaries at  and
and  . The boundary conditions are
. The boundary conditions are  at
at  . Since
. Since  by the definition of the stream-function, the boundary condition becomes
by the definition of the stream-function, the boundary condition becomes  . The equation of motion is
. The equation of motion is
 (1)
(1)
Suppose a rightward propagating wave exists within this waveguide,
 ,
,
then equation 1 becomes
 . (2)
. (2)
This is an eigenvalue problem which only has a simple solution when the stratification is linear (ie.  ). Equation (2) is then simplified to
). Equation (2) is then simplified to
 .
.
The solutions that satisfies both boundary conditions is

where  . The vertical wavenumber,
. The vertical wavenumber,  , is now quantized and only wave of a particular vertical structure exist. The vertical structure function,
, is now quantized and only wave of a particular vertical structure exist. The vertical structure function,  , phase speed,
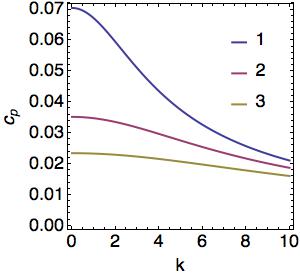
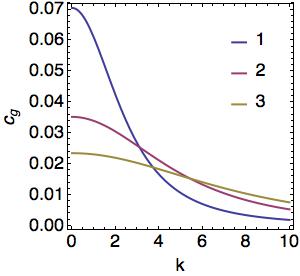
, phase speed,  , and group speed,
, and group speed,  for the first three modes are plotted below
for the first three modes are plotted below